TX to Ae

Ch 2. Contents

<< Part 3

Part 5 >>


2. Components and Materials: Part 4.
Contents:
2-11. Capacitance, capacitors and Insulators.
2-12. Measuring Permittivity.
2-13. Capacitor Impedance.		 2-14. Complex Permittivity.
2-15. Loss Tangent.
 

2-11. Capacitance, Capacitors and Insulators:
Just as resistance and inductance are distributed throughout any electrical circuit, so too is capacitance. Most readers will of course be familiar with stray-capacitance: the spurious coupling that exists between adjacent conductors and components, and self-capacitance: such as that which appears in parallel with a coil and reduces its effective inductance. We manage these phenomena: in the first instance by screening and a preference for low impedances in signal circuits, and in the second instance by learning to live with it and including it in our mathematical models. In all instances however, capacitance, whether wanted or spurious, is mediated by insulating materials; and it is a knowledge of the properties of such materials that must inform good RF practice. In particular, in power transmission applications, we need to understand the heating effect that occurs when insulators are subjected to strong time-varying (i.e., alternating) electric fields; since it is this which plays a large part in determining whether or not our coils, capacitors, transformers, and transmission lines will go up in flames.
     A capacitor, as should be understood by now, is nothing like a pure capacitance. It is instead, a device in which capacitance is engineered to some particular value (in Farads), and resistance and inductance are minimised to a degree suitable for the intended application. The basic objective is realised, in prototype form at least, by mounting two conducting plates in close proximity, with an electrical insulator of some description in between. In the context of capacitance, and also in the context of electromagnetic energy transmission, an insulating material is known as a dielectric. The term 'dielectric' means roughly: "opposed to", or "doesn't need" electricity; the general definition being: 'some medium in which a constant electrostatic field can be maintained without involving an appreciable supply of energy from external sources' [25].
     The capacitance of a capacitor is proportional to the overlap area of the plates A, and inversely proportional to the plate separation h, provided that a uniform electric field exists between the plates. The constant of proportionality is called the permittivity of the dielectric [6], and is given the symbol ε (Greek lower case 'epsilon'). The defining equation for capacitance is therefore:

C = ε A / h      Farads
If a vacuum exists between the capacitor plates, the permittivity is given the symbol ε0 ("epsilon nought"), and is known as the permittivity of free space. The internationally accepted value for ε0 is 8.854187818 pF/m (8.854 picoFarads per metre). If an insulating material other than vacuum is sandwiched between the plates (solid, liquid, or gas), the capacitance normally increases, and the amount by which the capacitance must be multiplied to account for the difference is called the relative permittivity, εr, of the intervening medium (also often given the symbol k). The capacitance equation then becomes:
C = ε0 εr A / h        Farads
2-11.1
i.e.,
ε = ε0 εr
εr (or k) is also called the dielectric constant of the medium; this being a form of irony since constancy is one attribute it does not have. It is a frequency and temperature dependent dimensionless complex number; and, as we shall see, it is closely related to the refractive index in optics, and the transmission-line velocity-factor in electronics, and it carries a wealth of information about the material to which it relates. The fact that relative permittivity is complex, of course, implies that capacitance is complex; but there is nothing esoteric in this statement: all it means is that the reactance of a capacitor is not completely imaginary, i.e., there is a real or resistive component in the reactance, this being due to dielectric losses. It does however beg the question: "why can't we simply represent the capacitor losses as a series resistance, and regard the dielectric constant as purely real?" There are two answers to this question: firstly, if the dielectric constant is real, then the definition of capacitance, C=εA/h, will only apply to DC circuits and vacuum capacitors, and so will generally be incorrect at radio or higher frequencies; and secondly, it transpires that the real and imaginary parts of of the dielectric constant cannot vary independently, because they represent different aspects of the same process, and so to separate them would be artificial. Before we delve into the inner workings of dielectrics however, we will finish with the basic definitions and show how to break down and analyse the various effects which contribute to the capacitor's impedance.

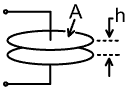2-12. Measuring Permittivity:
The measurement of permittivity at audio and radio frequencies is accomplished by measuring the impedance of a test capacitor and then correcting for unwanted contributions. If the measurement is made using a simple pair of conducting plates however, it will be found that the perfect proportionality of equation (2-11.1) is not exactly obeyed. In fact, the equation is most accurate when the area A is large, and the separation h is small; the reason being due to the non-uniformity of the electric field at the edges of the plates (see illustration right). The field-lines external to the capacitor somewhat resemble those of a bar-magnet, and although their contribution to the capacitance is small, it is not zero [26].

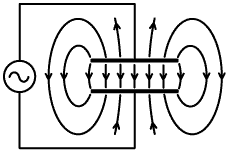     Note incidentally, that some of the field-lines must extend to infinity, and so the capacitor must be small in relation to the wavelength at the excitation frequency if it is not to exhibit appreciable radiation resistance. Note also, that the conduction currents flowing into and out of the capacitor will be confined to the surfaces of the plates at radio frequencies. This means that currents will enter the region between the plates from the edges, and energy will flow in the capacitor in the same way that it travels in a transmission line. A wave flowing across the capacitor will find a discontinuity (open-circuit) as it reaches the edge and will be reflected. The result will be a pattern of standing waves and a non-uniform field distribution between the plates unless the capacitor is small in relation to the wavelength at the excitation frequency.
     The solution to the edge-field (or fringing-field) problem when measuring permittivity is to encircle one of the capacitor plates with a guard-ring [10][18][25] (see right). During measurement, the guard-ring is maintained at the same potential (i.e., the same voltage) as the encircled plate, but forms no part of the measured capacitance. In this way, the fringing fields are associated only with the guard-ring, and the volume in which the capacitance is measured has a uniform electric field. The gap between the ring and the plate should be very small: a few percent of the capacitor spacing h; and the opposing plate should overlap the full area of the plate and guard-ring.


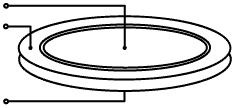Guard-ring capacitor.
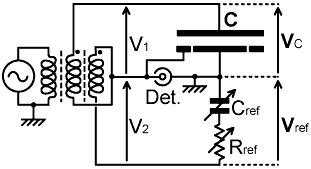
     The guard-ring can be maintained at the same potential as the associated capacitor plate by using a bridge circuit, one possible configuration being shown in the illustration below:


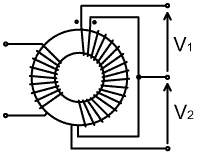The arrangement shown is known as a transformer ratio-arm bridge or TRAB, and makes use of a bifilar-wound transformer to produce two voltages in series (i.e., V1 and V2) that are exactly identical in both magnitude and phase. A proper physical interpretation of the transformer symbol is given on the right, the dots indicating the points where the associated windings may be considered to start or finish. Such a transformer is nowadays best constructed using a small ferrite ring or 'toroid', with the excitation winding (the one connected to the generator) spaced a little away from the bifilar pair to prevent stray capacitance from upsetting the electrical symmetry. The excitation source is an RF signal generator, and the detector (connected to the port labelled "Det.") is typically a radio receiver tuned to the generator frequency. In operation, the reference resistor Rref and the reference capacitor Cref are adjusted until the signal at the detector is minimised; in which case the voltage across the test capacitor (VC) is identical in magnitude and phase to the voltage across the impedance formed by Rref and Cref, (i.e., Vref) and the bridge is said to be 'balanced'. When the bridge is balanced, the impedance of the test capacitor is exactly equal to Rref+jXCref, and since the voltage across the detector is then effectively zero, the guard-ring is at exactly the same electrical potential as the plate it encircles and any errors due to fringing-fields are rendered negligible.
     A bridge measurement of course, requires a calibrated non-inductive non-capacitive variable resistor, and a calibrated non-resistive, non-inductive variable capacitor; neither of which are physically realisable. Extremely accurate measurements therefore require corrections for the imperfections of the reference components; i.e., we have to know the impedances of the reference components (and the connecting wires) at the test frequency, and we obtain the true reference impedance by adding these together. There also exists a correction formula, derived by Maxwell himself, for the very small non-uniformity of field which results from the gap between the earthed-plate and the guard-ring [25]. The uncorrected system however, while unlikely to impress the scientists who work for national standards bodies, is capable of engineering measurements, and will suffice to illustrate our point.

See also: [Heerens & Vermeulen 1975, Capacitance of guard-ring capacitors . . .]
[Moon & Sparks 1948, RP1935]

2-13. Capacitor Impedance:
If a capacitor is perfect, the current that flows in and out of its terminals is exactly 90° out of phase with the applied voltage, i.e., the impedance of the capacitor is entirely imaginary. We cannot expect perfection however; and so the simplest capacitor model that we can use must consist of an ideal capacitor in series with a small amount of resistance. Such a model is shown below; where Res is the equivalent series resistance (ESR), and Ces is the effective capacitance when the impedance of the capacitor is expressed in series (R+jX) form.

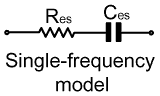Res = equivalent series resistance (ESR)

Ces = series-equivalent capacitance.
This model is only applicable to a single frequency, because both Ces and Res vary with frequency. We expect the capacitance to vary of course, because there must be a magnetic field associated with any current flowing in the capacitor and its connecting wires; and the resulting inductive reactance must cancel some of the capacitive reactance, making the capacitance appear to increase as the frequency is increased. There will, incidentally, also be a resonant frequency, above which the capacitor will behave as an inductor; and so a single-frequency model that depicts the device as a capacitor is only applicable below the resonant frequency. We also expect a resistive component, part of it being attributable to the connecting wires and the metal plates, with a frequency variation due to the skin effect. When a material other than a vacuum is placed between the plates however, there is normally an increase in both the capacitance and the resistance, and these new elements have frequency (and temperature) dependencies of their own. To study dielectrics we must therefore separate these latter quantities from ordinary circuit effects, and in order to do so we will extend our capacitor model as shown below:

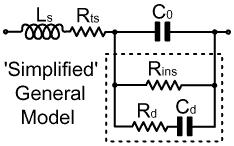Ls = series inductance
Rts = 'true' (conductor) series resistance (TSR)
C0 = capacitance 'without' dielectric.
Rins = insulation leakage resistance
Rd = dielectric losses
Cd = additional capacitance due to the dielectric

The series inductance Ls is due to the plates and connecting wires, and its inclusion in the model allows true capacitance to be isolated by making measurements at several frequencies. Likewise, the so-called 'true series resistance' is due to the plates and connecting wires, and its contribution to the impedance at a particular frequency can be determined by operating the capacitor without a dielectric (i.e., with a vacuum dielectric, or some approximation to it such as air). The capacitance in the absence of a dielectric is represented by C0; and the three components inside the dotted box are the properties that only appear when a dielectric is present. Rins is the insulation leakage resistance, Rd is the dielectric loss, and Cd is the additional capacitance of the dielectric. The model is called a 'simplified' general model because it lacks physical realism in several important respects: the main ones being that it does not include any transmission-line effects, and the reactance of the dielectric cannot be represented as a single series R-C combination (Rd+jXCd); but requires, in principle (but fortunately not always in practice) an LCR network for every single mechanical, atomic, and molecular process that can occur within the material. This story will unfold in the discussion to follow; but we will first familiarise ourselves with this basic model and its relationship to the single-frequency model given earlier.
     The insulation leakage resistance, Rins, arises from the fact that no insulator (excepting vacuum) is completely perfect. It is not strictly a dielectric process because it relates to electrical conduction; but it is usually lumped into the dielectric losses because the effort of trying to separate it is neither worthwhile nor theoretically necessary. The types of insulators used in (properly designed) radio and electrical installations have resistivities in the range 1010 - 1019 Ωm (compared to a few tens of nΩm for conductors), and so, provided that we keep our equipment clean and dry, Rins is likely to be too large to make a measurable contribution to the system losses. A sample of polyethylene (polythene), for example, might have a resistivity of about 1016 Ωm. If we clamp a 1 mm thick sheet of this material between the plates of a test capacitor having an overlap area of 0.01 m² (112.8 mm diameter), the leakage resistance (assuming that the plates make good contact) will be:
Rins = ρh/A = 1016 ×10-3 / 0.01 = 1015 Ω = 1000 TΩ
In practice, the plates will not make good contact, and the resistivities of such awkward substances have to be measured by vacuum deposition of metal electrodes directly onto the material surfaces. In general, the only non-defective capacitors for which leakage resistance can be measured using commonplace test equipment are electrolytic capacitors.
     The diagram below shows a notional transformation, which takes us from the simplified general model to the series equivalent model from which the impedance of the capacitor is derived:


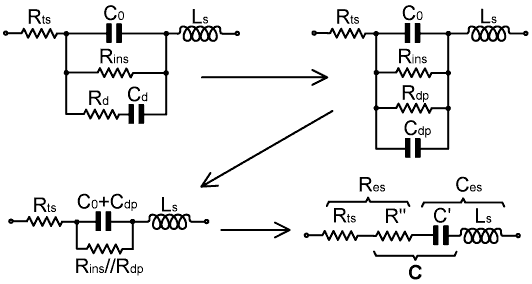
The point of this illustration is to show that all of the translations between models are obtained purely by application of the series-parallel and parallel-series transformations described in [AC Theory, Sections 18 and 19]. We do not necessarily have cause to carry out such transformations explicitly, but it is important to understand that all of the models are equivalent at a particular frequency. In this case, we end up with the equivalent series resistance (ESR) of the capacitor, Res, being split into the true (conductor) series resistance Rts, and a dielectric loss component R" ("R double prime"). Similarly, the series-equivalent capacitance Ces is split into the circuit inductance Ls and a real capacitance C' ("C prime"). The reactance of the network will be capacitive provided that -XC' is always larger than XLs, a situation we must arrange if we want to persist in calling the device a capacitor. Now the important part: notice that if the dielectric is removed, Rins, Rd, and Cd in the initial model all disappear; and if we compare what is left to the final model, we see that the series combination R" and C' in the final model replaces C0 when a dielectric is inserted. Hence the network consisting of R" in series C' is the true capacitance C; and capacitance, as was mentioned previously, is in general complex.

2-14. Complex permittivity:
The general capacitance equation was given earlier as:
C = ε A / h
    = ε0 εr A / h    
We know that if the dielectric is removed, we obtain a capacitance:
C0 = ε0 A / h
but when a dielectric filling is inserted, C must be represented as a network consisting of a resisance in series with a capacitance. Since capacitance is also defined in terms of impedance; the only way in which we can force the definition of capacitance to be true in general is by assuming that permittivity contains an element that acts at +90° relative to the -90° of a pure capacitive reactance; i.e., permittivity has an element that gives rise to a resistive (0°) component in the capacitor's impedance. This, of course, requires that permittivity is complex, and it is customary to write it as:
ε = ε' -jε"
(the choice of sign is that which makes the resulting resistive component positive).
Now, since vacuum capacitors do not have dielectric losses (i.e., ε0 is real), we can further identify the dielectric constant εr as the complex entity, i.e.:
ε = ε0 εr
   = ε0 ( εr' -jεr" )
Hence:
εr = εr' -jεr"
which has a magnitude:
|εr| = √[(εr')² + (εr")²]

We are now in a position to write the expression for the true reactance of a capacitor (i.e., the reactance after correction for the circuit elements Ls and Rts) as:
XC = -1/( 2πf C )
     = -1/[ 2πf ε0 ( εr' -jεr" )( A / h ) ]
but note that the term ε0A/h is just the capacitance of the capacitor without a material dielectric, i.e., it is C0, hence:
XC = -1/[ 2πf C0 ( εr' -jεr" ) ]
and -1/(2πf C0) is XC0, hence:
XC = XC0 / ( εr' -jεr" )
Now, to make the denominator real, so that we may separate the reactance into its real and imaginary parts; we multiply the numerator and the denominator by the complex conjugate of the denominator, i.e.:
XC = XC0 ( εr' + jεr" ) / [( εr' -jεr" )( εr' + jεr" )]
hence, bearing in mind that j²=-1:
XC = XC0 ( εr' + jεr" ) / [(εr')² + (εr")²]
As is always the case when this operation is performed, the new denominator is just the square of the magnitude of the original complex denominator, i.e., in this case, [(εr')²+(εr")²]=|εr|², hence:
XC = XC0 ( εr' + jεr" ) / |εr|²
The impedance of the 'true' capacitance is then:
ZC = jXC
     = jXC0 ( εr' + jεr" ) / |εr|²
     = XC0 ( -εr" + jεr' ) / |εr|²
Now recall that we expressed the true capacitance as a network consisting of a capacitance C' in series with a resistance R". Hence:
XC' = XC0 εr' / |εr|²
and
R" = -XC0 εr" / |εr|²
2-14.1
(R" is positive because XC0 is negative).

2-15. Loss Tangent:
Shown below is a set of phasor diagrams that summarises our deconstruction of the capacitor model so far. The loss resistances in each case are considerably exaggerated for the purpose of clarity.

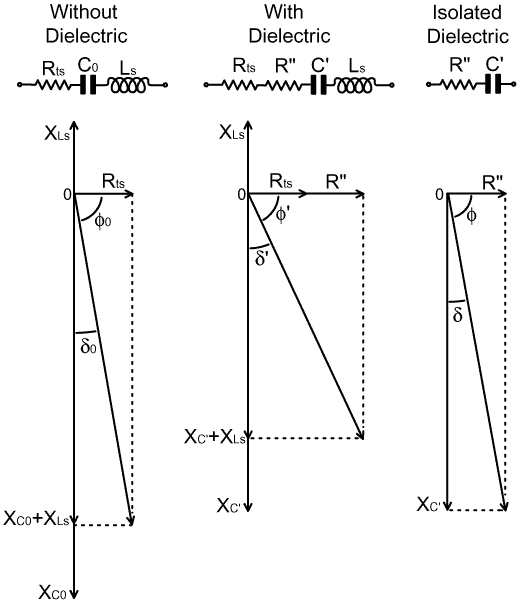
For the capacitor without a material dielectric, the true capacitance C0 can, in principle, be obtained from the capacitor dimensions. It is then possible to obtain the series inductance Ls from the discrepancy between the observed and the theoretical reactance, and the conductor resistance Rts from the real part of the impedance. Measurements must be made at several frequencies and compiled into a graph of corrections for the test setup because both Ls and Rts vary with frequency. Strictly, for this characterisation, the capacitor should be operated in a vacuum; but the dielectric constant of air is well known (εr' = 1.0005361 at 1bar and 20°C [8]) and its losses are extremely small (i.e., we can usually assume that εr" =0), and so a correction for the air can easily be applied. If extremely accurate measurements are to be made, the reference air should be dry; i.e., the capacitor should be operated in a closed vessel in the presence of a desiccant such as silica-gel, the dielectric constant of air at 20°C rising to 1.00066 for 60% relative humidity [8a]. For engineering purposes however, knowledge of relative permittivity to three decimal places is usually adequate, in which case, ordinary room air can be assumed to have εr'=1.0007.
     When a dielectric substance is inserted into the capacitor; note that the capacitance generally increases, and so the capacitive reactance is reduced. The phasor diagram in this case relates just as well to practical capacitors as it does to dielectric test equipment; and so it is appropriate here to point-out that the performance figures supplied by capacitor manufacturers relate to the capacitor as a whole, i.e., the conductor TSR and the series inductance are included in the impedance specified for a particular frequency. Also note, as mentioned earlier, that the inductive reactance subtracts from the capacitive reactance, making the the capacitance appear larger than its DC value.
     The phasor diagram for an isolated dielectric, of course, cannot be obtained directly. It is instead determined by subtracting Rts and jXLs from the impedance of a test capacitor, using calibration data for the frequency at which the test is conducted. It is nevertheless a true characterisation of the dielectric; and presuming that the stated frequency range covers our intended application, it provides us with a qualitative and, as we shall see later, a quantitative measure of the suitability of an insulating material for operation in the presence of alternating electric fields.
     Now notice, that in each of the phasor diagrams there is marked an angle δ (delta). This is known as the loss angle, and is a standard and widely used measure of capacitor and insulator performance. Its value is simply 90°-φ, where φ is the phase angle of the associated impedance. The quantity normally quoted however is Tanδ, which is known as the loss tangent. Tanδ is zero for a perfect insulator or capacitor and increases as the losses increase. Only vacuum has Tanδ=0, although for common gases (N2, O2, CO2, Ar, etc.) it can be taken to be immeasurably small at radio frequencies. Looking at the phasor diagrams above, observe that δ is an angle of a right-angled triangle, with a loss resistance as its opposite side, and a reactance magnitude as its adjacent side (i.e., we must take the reactance side of the triangle to be positive, even though capacitive reactance is negative). Therefore:
Tanδ = loss resistance / |reactance|
or, alternatively:
Tanδ = - loss resistance / capacitive reactance
because, for a pure capacitive reactance:
|X| = -X

For a complete capacitor
Tanδ' = ( Rts + R" ) / | XC' + XLs |
but recall that Rts+R"=Res, the equivalent series resistance or ESR; and XC'+XLs=XCes, the series equivalent reactance. Hence, for the purpose of interpreting the figures quoted in capacitor data sheets:
Tanδ' = Res / |XCes|
i.e.:
Tanδ' = -Res / XCes
(the prime is only included for the purpose of distinguishing the various quantities involved in this discussion, and does not appear in component data sheets).

For an insulator
Tanδ = -R" / XC'
Now recall that we have already derived expressions for R" and XC' in terms of the complex permittivity (equations 2-14.1):
These are: 
XC'=XC0εr'/(|εr|²) and R"=-XC0εr"/(|εr|²). 
Hence:
Tanδ = εr" / εr'
2-15.1
This is a profound result, because the equation does not contain any length, area, capacitance, or reactance terms. It tells us that the Tanδ value for a particular insulator is completely independent of the physical shape or value of the capacitor that was used to determine it. This means that, however a capacitance is produced with a particular dielectric, the value of Tanδ at a particular frequency, once separated from the other circuit effects, is determined entirely by the dielectric. It does not matter what form the capacitance takes: it might be the distributed capacitance in a transmission line, it might be a coil-former or wire insulation involved in the self-capacitance of a coil, it might be an insulating pillar, it could even be a capacitor; Tanδ is the loss tangent for that capacitance. In terms of losses therefore, there is only one basic selection criterion for insulating materials to be used in radio-frequency applications; we want Tanδ to be as small as possible.
     In modern technical literature, dielectric properties are usually stated in terms of εr' and Tanδ. If, for any reason, εr" is required, it can be obtained from equation (2-15.1) above, i.e.:
εr" = εr' Tanδ
also, since εr = εr' -jεr"
εr = εr' ( 1 -j Tanδ )
In older literature however, dielectric losses are often stated in terms of power factor (PF). You might recall from the previous chapter, that the power factor of an impedance is the cosine of the phase angle, i.e., PF=Cosφ. Since δ=90-φ, then power factor is also equal to Sinδ. Tanδ and Sinδ are very nearly equal if the angle δ is small; but if the power factor is greater than about 0.1, it is advisable take the arcsin (inverse sine) to obtain δ, then calculate Tanδ, i.e.:
Tanδ = Tan[ Arcsin(PF) ]
The result should be rounded to the same number of decimal places as was given for the power factor. In books and papers not adhering to the complex permittivity convention, "dielectric constant", "k", or "specific inductive capacity" is equivalent to εr'.
     In general, for use in the presence of strong RF fields, a dielectric should be considered to be acceptable only if it produces a loss angle of substantially less than 1°, i.e., a phase angle between 89 and 90°. Tanδ=0.01 corresponds to δ=0.57°, and so is a figure to bear in mind as a reasonable upper limit for suitability. Properties of various dielectrics are listed later in this chapter; but before we examine the technical data we will arm ourselves for the purpose of interpreting it by taking a look at the processes that occur inside dielectric materials.

Part 5 >>

© D W Knight 2007.
Last edited:  2019-May-26
David Knight asserts the right to be recognised as the author of this work.
TX to Ae
Ch 2. Contents
<< Part 3
Part 5 >>